AppTail AppTail.

Modular Arithmetic

Calculate in remainders mod n

Разработчик: Benjamin Burton
Посмотреть в магазине
Открыть
Скачивания
Открыть
Доход

Описание

A calculator for arithmetic modulo N. It lets you choose a fixed modulus, and then make lots of calculations without having to press a "mod" button again and again. It also:
- follows the order convention;
- supports arbitrarily large numbers;
- performs fast modular division and exponentiation;
- can show a full transcript of your calculation.
Modular arithmetic is a "calculus of remainders". It features throughout mathematics and computer science, and has applications from cryptography to barcodes to music.
The basic idea is that you choose a modulus N, and then reduce every number to one of the integers 0,1,2,...,N−1 according to what remainder it leaves when dividing by N.
For example, using a modulus of 17:
40 ≡ 6 (since 40 ÷ 17 leaves a remainder of 6);
17 ≡ 0 (since 17 ÷ 17 leaves no remainder at all).
Arithmetic follows these same rules. Still using a modulus of 17:
15 + 7 ≡ 5 (since 22 ≡ 5);
3 × 9 ≡ 10 (since 27 ≡ 10);
5 ^ 3 ≡ 6 (since 125 ≡ 6).
Subtraction and division behave in a way that complements addition and multiplication:
−1 ≡ 16 (since 16 + 1 = 17 ≡ 0);
1/2 ≡ 9 (since 9 × 2 = 18 ≡ 1);
4 - 7 ≡ 14 (since 14 + 7 = 21 ≡ 4);
7 ÷ 3 = 8 (since 8 × 3 = 24 ≡ 7).
There are no negative numbers or fractions: like −1 and 7 ÷ 3 in the examples above, these are also reduced to one of 0,1,...,N−1.
As usual, you cannot divide by zero. You also cannot divide if the right hand side has any common factors with the modulus. If we change our modulus to 10, then the following operations all generate errors:
3 ÷ 20 (since 20 ≡ 0);
7 ÷ 8 (since 8 and 10 have a common factor of 2).
Integers can be arbitrarily large. For instance, if we set our modulus to 2305843009213693951 (a Mersenne prime), then:
5 ^ 2305843009213693950 ≡ 1 (by Fermat's little theorem).
The code is written carefully, and is backed up by a thorough suite of 186 automated tests.
This app supports external keyboards, Siri Shortcuts, and (on iPad) Slide Over, Split View, and multiple windows.
Скрыть Показать больше...

Скриншоты

Modular Arithmetic Частые Вопросы

  • Приложение Modular Arithmetic бесплатное?

    Modular Arithmetic не является бесплатным (стоимость составляет 9.99), однако оно не содержит встроенных покупок или подписок.

  • Является ли Modular Arithmetic фейковым или мошенническим?

    Недостаточно отзывов для надежной оценки. Приложению нужно больше отзывов пользователей.

    Спасибо за ваш голос

  • Сколько стоит Modular Arithmetic?

    Цена Modular Arithmetic составляет 9.99.

  • Сколько зарабатывает Modular Arithmetic?

    Чтобы получить оценку дохода приложения Modular Arithmetic и другие данные AppStore, вы можете зарегистрироваться на платформе мобильной аналитики AppTail.

Оценки пользователей

5 из 5

1 оценок в Румыния

5 star
1
4 star
0
3 star
0
2 star
0
1 star
0
История оценок

Modular Arithmetic Отзывы Пользователей

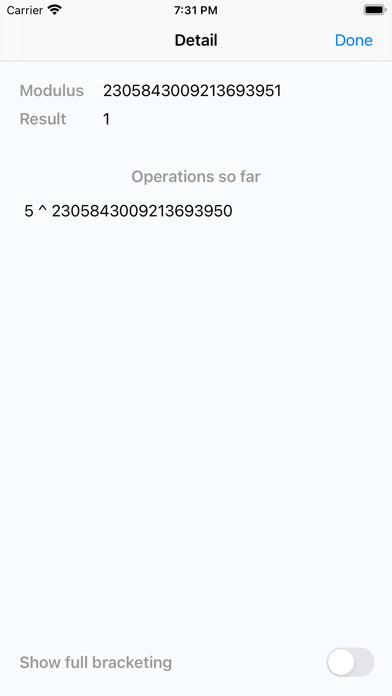
nice idea!

einffall on

Румыния

it would be even more useful if we would see a more descriptive result: 1. written in that base Like, with modulo 26, we have 729 = 1*26^2 + 2*26 + 1 or (1 2 1) 2. together with quotient 729 = 28*26 + 1 i would love to see these 2 forms, what do you think?

Показать больше

Оценки

История позиций в топах
История рейтингов пока не доступна
Позиции в категории
Приложение еще не было в топах

Modular Arithmetic Конкуренты

Name
It. Diction
Times Tables - Math
Create beautiful patterns
Ladhe's Primes
Prime Numbers
LCM GCD Prime Factor Math
Calculator factorize numbers
Fibs and Primes
Gear Design
Goldbach's Conjecture
SQL AI
Learn SQL
NetTrainer
Pythagoras Interactive
Показать больше

Modular Arithmetic Установки

30дн.

-1

Установки - посмотреть данные

Modular Arithmetic Доход

30дн.

$-1

Доход - посмотреть данные

Modular Arithmetic Доходы и Загрузки

Получите ценные инсайты о производительности Modular Arithmetic с помощью нашей аналитики.
Зарегистрируйтесь сейчас, чтобы получить доступ к статистика загрузок и доходов и многому другому.

Информация о приложении

Категория
Education
Разработчик
Benjamin Burton
Языки
English
Последнее обновление
4.1 (1 месяц назад )
Выпущено
Aug 17, 2013 (11 лет назад )
Также доступно в
Австралия, Соединенные Штаты, Норвегия, Румыния, Казахстан, Перу, Новая Зеландия, Нидерланды, Малайзия, Мексика, Ливан, Польша, Кувейт, Республика Корея, Япония, Италия, Индия, Пакистан, Израиль, Португалия, Россия, Саудовская Аравия, Швеция, Сингапур, Таиланд, Турция, Тайвань, Украина, Вьетнам, Южно-Африканская Республика, Дания, Аргентина, Австрия, Азербайджан, Бельгия, Бразилия, Беларусь, Канада, Швейцария, Чили, Китай, Колумбия, Чехия, Германия, ОАЭ, Доминиканская Республика, Алжир, Эквадор, Египет, Испания, Финляндия, Франция, Великобритания, Греция, Гонконг (САР), Венгрия, Индонезия, Ирландия
Обновлено
2 дня назад
AppTail.

Product

Ресурсы

Contact